Read about gravitational potential in the right column before looking at the exercises.
The simulation contains two stars. The mass and radius of the second star are so small that in practice there is only one star.
Exercises
-
Click OK on the parameter page, but don't start the simulation. Click on the Gravitational Field tab. Study the equipotential lines.
Click on neighboring lines and note that the potential difference between lines is constant.
When looking at the lines you may get the impression of looking down into a "well". Therefore we often say that the star is at the bottom of a potential well. Here is a 3D graph of a potential well: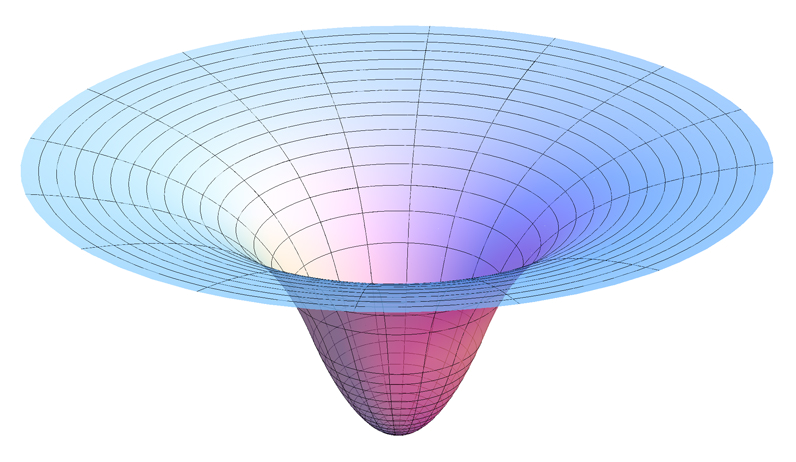
-
Calculate the gravitational potential at the surface of the star S1.
Click close to the surface and compare with your calculated value. -
Change the mass of S2 to 1E30 kg and the radius to 5E5 km.
Click OK and study the equipotentials.
Try to calculate the potential at the midpoint of a line connecting the two stars and compare with the value you can read by clicking in the Field window. -
Choose the menu item Miscellaneous, Advanced and check Field Lines.
Click on the Field tab. Note that the field line through any point is always perpendicular to the equipotential line through the same point. Try to explain this fact. - Zoom out a few times and watch the equipotential lines. Try to explain that they get more circular with increasing distance from the stars.